Chapter 3 Binomial Theorem-PDF Free Download
Class 11 Maths Chapter 8 Binomial Theorem Binomial Theorem for Positive Integer If n is any positive integer, then This is called binomial theorem. Here, nC 0, nC 1, nC 2, , nn o are called binomial coefficients and nC r n! / r!(n – r)! for 0 r
Part One: Heir of Ash Chapter 1 Chapter 2 Chapter 3 Chapter 4 Chapter 5 Chapter 6 Chapter 7 Chapter 8 Chapter 9 Chapter 10 Chapter 11 Chapter 12 Chapter 13 Chapter 14 Chapter 15 Chapter 16 Chapter 17 Chapter 18 Chapter 19 Chapter 20 Chapter 21 Chapter 22 Chapter 23 Chapter 24 Chapter 25 Chapter 26 Chapter 27 Chapter 28 Chapter 29 Chapter 30 .
TO KILL A MOCKINGBIRD. Contents Dedication Epigraph Part One Chapter 1 Chapter 2 Chapter 3 Chapter 4 Chapter 5 Chapter 6 Chapter 7 Chapter 8 Chapter 9 Chapter 10 Chapter 11 Part Two Chapter 12 Chapter 13 Chapter 14 Chapter 15 Chapter 16 Chapter 17 Chapter 18. Chapter 19 Chapter 20 Chapter 21 Chapter 22 Chapter 23 Chapter 24 Chapter 25 Chapter 26
11 Permutations, Combinations, and the Binomial Theorem Key Terms fundamental counting principle factorial permutation combination binomial theorem on heorem Combinatorics, a branch of discrete mathematics, can be defined as the art of counting. Famous links to combinatorics include Pascal’s triangle, the magic square,
1 Chapter 5 Test Review Pre-AP Algebra II – Chapter 5 Test Review Standards/Goals: A.1.b./A.APR.5.: o I can expand a binomial using Pascal’s Triangle. o I can use the binomial theorem to expand a binomial. A.1.c./F.1.b.:I can factor a qua
Stokes’ and Gauss’ Theorems Math 240 Stokes’ theorem Gauss’ theorem Calculating volume Stokes’ theorem Theorem (Green’s theorem) Let Dbe a closed, bounded region in R2 with boundary C @D. If F Mi Nj is a C1 vector eld on Dthen I C Mdx Ndy ZZ D @N @x @M @y dxdy: Notice that @N @x @M @y k r F: Theorem (Stokes’ theorem)
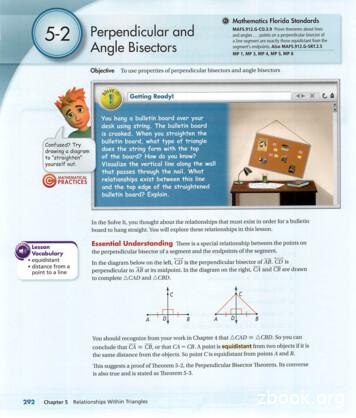
Nov 19, 2018 · Theorem 5-4 Angle Bisector Theorem Theorem If a point is on the bisector of an angle, then the point is equidistant from the sides of the angle. If. . . QS bisects PQR, 1 QP, and SR 1 QR P, S Then. SP SR You will prove Theorem 5-4 in Exercise 34. Theorem 5-5 Converse of the Angle Bisector Theorem Theorem
Triangle Sum Theorem Exterior Angle Theorem Third Angle Theorem Angle - Angle- Side Congruence Theorem non Hypotenuse- Leg Theorem the triangles are congruent Isosceles Triangle Theorem Perpendicular Bisector Theorem If a perpendicular line is
normal curve can approximate a binomial distribution with n 10 and p q 1/2. Figure 4-5 illustrates a case where the normal distribution closely approximates the binomial when p is small but the sample size is large. Figure 4-4. Binomial distribution for p 0.5 and n 10. Figure 4-5. Binomial distribution for p 0.08 and n 100.
(Quasi)-Poisson vs. Negative Binomial regression Theoretically, one could specify a Quasi-Negative Binomial and/or a Zero-in ated Negative Binomial model using the same machinery we have already introduced, but I have never seen these used in practice. Negative Binomial GLMs tend to handle zero-in ation reasonably well,
Quasi-likelihood Negative binomial The negative binomial distribution The negative binomial distribution has other uses in probability and statistics, but for our purposes we can think about it as arising from a two-stage hierarchical process: Z Gamma( ; ) YjZ Poisson( Z) The marginal distribution of Y is then negative binomial, with E(Y)
number of plants in the plot. It is better to treat these counts as having a binomial distribution rather than a Poisson or negative binomial. Binomial. Binomial data are discrete positive integers between 0 and n. It is the standard distribution for the number of successes from n independent trials with only two outcomes.
Poisson (ZIP), and zero-inflated negative binomial (ZINB) distributions. Then we try to fit each of these data sets with the four corresponding count regression models. The Poisson and negative binomial data sets are generated using the same conditional mean: i D e1C0:3x1iC0:3x2i (2) In addition, the negative binomial model further uses the .
Probability Distribution. Mean of a Discrete Random Variable. Standard Deviation of a Discrete Random Variable. Binomial Random Variable. Binomial Probability Formula. Tables of the Binomial Distribution. Mean and Standard Deviation of a Binomial Random Variable. Poisson Random Variable. Poisson Probability Formula. Hypergeome tric Random Variable.
DEDICATION PART ONE Chapter 1 Chapter 2 Chapter 3 Chapter 4 Chapter 5 Chapter 6 Chapter 7 Chapter 8 Chapter 9 Chapter 10 Chapter 11 PART TWO Chapter 12 Chapter 13 Chapter 14 Chapter 15 Chapter 16 Chapter 17 Chapter 18 Chapter 19 Chapter 20 Chapter 21 Chapter 22 Chapter 23 .
3.4. Sequential compactness and uniform control of the Radon-Nikodym derivative 31 4. Proof of Theorem 2.15 applications 31 4.1. Preliminaries 31 4.2. Proof of Theorem 1.5 34 4.3. Proof of Theorem 1.11 42 4.4. Proof of Theorem 1.13 44 5. Proof of Theorem 2.15 46 6. Proof of Theorem 3.9 48 6.1. Three key technical propositions 48 6.2.
Numerical Methods for Option Pricing in Finance Chapter 2: Binomial Methods and the Black-Scholes Formula 2.1 Binomial Trees One-period model of a financial market We consider a financial market consisting of a bond Bt B(t), a stock St S(t), and a call-option Ct C(t), where the trade is only possible at time t 0 and t t. Assumptions:
2 12 8 10 6 3 2 4 5 1 11 7 9 Permutations, Combinations and the Binomial Theorem October 27, 2011 2 / 24. Remark A sorted sequence (array) is a sequence with no inversions. Thus the goal of a sorting procedure is to remove all inversions from the given sequence. Question
MHR 978-0-07-0738850 Pre-Calculus 12 Solutions Chapter 11 Page 1 of 77 Chapter 11 Permutations, Combinations, and the Binomial Theorem Section 11.1 Permutations Section 11.1 Page 524 Question 1
About the husband’s secret. Dedication Epigraph Pandora Monday Chapter One Chapter Two Chapter Three Chapter Four Chapter Five Tuesday Chapter Six Chapter Seven. Chapter Eight Chapter Nine Chapter Ten Chapter Eleven Chapter Twelve Chapter Thirteen Chapter Fourteen Chapter Fifteen Chapter Sixteen Chapter Seventeen Chapter Eighteen
18.4 35 18.5 35 I Solutions to Applying the Concepts Questions II Answers to End-of-chapter Conceptual Questions Chapter 1 37 Chapter 2 38 Chapter 3 39 Chapter 4 40 Chapter 5 43 Chapter 6 45 Chapter 7 46 Chapter 8 47 Chapter 9 50 Chapter 10 52 Chapter 11 55 Chapter 12 56 Chapter 13 57 Chapter 14 61 Chapter 15 62 Chapter 16 63 Chapter 17 65 .
HUNTER. Special thanks to Kate Cary. Contents Cover Title Page Prologue Chapter 1 Chapter 2 Chapter 3 Chapter 4 Chapter 5 Chapter 6 Chapter 7 Chapter 8 Chapter 9 Chapter 10 Chapter 11 Chapter 12 Chapter 13 Chapter 14 Chapter 15 Chapter 16 Chapter 17 Chapter
Chapter 3 Chapter 4 Chapter 5 Chapter 6 Chapter 7 Chapter 8 Chapter 9 Chapter 10 Chapter 11 Chapter 12 Chapter 13 Chapter 14 Chapter 15 Chapter 16 Chapter 17 Chapter 18 Chapter 19 Chapter 20 . Within was a room as familiar to her as her home back in Oparium. A large desk was situated i
12. B.Chattopadhyay & P.C.Rakshit; Fundamental of Electrical circuit theory; S Chand 13. Nilson & Riedel , Electric circuits ;Pearson List of experiments (Expandable): 1. To Verify Thevenin Theorem. 2. To Verify Superposition Theorem. 3. To Verify Reciprocity Theorem. 4. To Verify Maximum Power Transfer Theorem. 5. To Verify Millman’s Theorem.
Figure 7: Indian proof of Pythagorean Theorem 2.7 Applications of Pythagorean Theorem In this segment we will consider some real life applications to Pythagorean Theorem: The Pythagorean Theorem is a starting place for trigonometry, which leads to methods, for example, for calculating length of a lake. Height of a Building, length of a bridge.File Size: 255KB
SAS Similarity Theorem 7. The postulate or theorem that can be used to prove that the two triangles are similar is _. a. SAS Similarity Theorem b. ASA Congruence Theorem c. SSS Similarity Theorem d. AA Similarity Postulate. 2 8. Given: PQ BC. Find the length of AQ. a. 11 b. 9 c. 13 d. 6 9. Find the value of x to one decimal place.
Maximum Power Transfer Theorem Electric Circuit 72 Maximum Power Transfer Theorem. 18/06/50 Electric Circuit 73 Maximum Power Transfer Theorem Electric Circuit 74 Maximum Power Transfer Theorem. 18/06/50 Electric Circuit 75 Maximum Power Transfer Theorem Electric Ci
Grade 8 Pythagorean Theorem (Relationship) 8.SS.1 Develop and apply the Pythagorean theorem to solve problems. 1. Model and explain the Pythagorean theorem concretely, pictorially, or by using technology. 2. Explain, using examples, that the Pythagorean theorem applies only to right triangles. 3. Determine whether or not a triangle is a right .
Some Applications of the Bounded Convergence Theorem for an Introductory Course in Analysis JONATHAN W. LEWIN Kennesaw College, Marietta, GA 30061 The Arzela bounded convergence theorem is the special case of the Lebesgue dominated convergence theorem in which the functions are assumed to be Riemann integrable. THE BOUNDED CONVERGENCE THEOREM.
CADverter v23.2 for JT-Catia Composer Page 8 Theorem Solutions 2020 Using the Catia Composer Product Once the Theorem JT-Catia product has been installed, the options to select the "Jt Theorem (.jt) format and the "PLMXML Theorem (.plmxml)" format will be added to the "Open" "Files of type" list.
Poisson-like assumptions (that we call the quasi-Poisson from now on) or a negative binomial model. The objective of this statistical report is to introduce some concepts that will help an ecologist choose between a quasi-Poisson regression model and a negative binomial regression model for overdispersed count data.
Poisson versus Negative Binomial Regression Randall Reese Utah State University rreese531@gmail.com February 29, 2016 Randall Reese Poisson and Neg. Binom. Handling Count Data The Negative Binomial Distribution Other Applications and Analysis in R References Overview 1 Handling Count Data
MCQ 8.3 Nature of the binomial random variable X is: (a) Quantitative (b) Qualitative (c) Discrete (d) Continuous MCQ 8.4 In a binomial probability distribution, the sum of probability of failure and probability of success is always: (a) Zero (b) Less than
Oct 26, 2014 · Stochastic Calculus for Finance I: The Binomial Asset Pricing Model Solution of Exercise Problems Yan Zeng Version 1.1, last revised on 2014-10-26 Abstract This is a solution manual for Shreve [6]. If you find any typos/errors or have any comments, please email me at zypublic@hotmail.edu. Contents 1 The Binomial No-Arbitrage Pricing Model 2
difference of squares, i.e. the front binomial. We get ( )( ) ( )( )( ) Since each binomial is factored completely, this is our answer. Note: If you are trying to factor a binomial and you have the option of both the difference of squares and the difference of cubes, always factor the differe
each term in its simplest form. (Total 4 marks) C2 Sequences & Series: Binomial Expansion Edexcel Internal Review 2 5. (a) Find the first 4 terms, in ascending powers of x, of the binomial expansion of (1 ax)10, where a is a non-zero consta
Created by T. Madas Created by T. Madas Question 25 (*** ) a) Determine, in ascending powers of x, the first three terms in the binomial expansion of ( )2 3 x 10. b) Use the first three terms in the binomial expansion of ( )2 3 x 10, with a suitable value for x, to find an approximation for 1.97 10. c) Use the answer of pa
of a sum and difference of two terms, square of a binomial, cube of a binomial and product of special case of multiplying a binomial with a trinomial factor completely different types of polynomials (polynomials with common monomial factors, a difference of two squares, sum and difference of two
Binomial option model The binomial option pricing model is an iterative solution that models the price evolution over the whole option validity period. For some types of options, such as the American options, using an iterative model is the only choice since there is no known closed-form solution that predicts price over time.
Moreover Binomial model is more accurate and converges faster than Monte Carlo method when pricing European options. KEYWORDS: Accuracy, Binomial Model, European option, Monte Carlo Method Mathematics Subject Classification: 6 5C05, 65C30, 91G60, 60H30, 65N06, 78M31 INTRODUCTION Option pricing is a major accomplishment of modern finance.