1.6 Angle Pair Relationships
Angle PairRelationships
Angle Pair RelationshipEssential QuestionsHow are special angle pairsidentified?
Straight AnglesOppositerays are two rays that are part of a the same line and haveonly their endpoints in common.YXZopposite raysXY and XZ are .The figure formed by opposite rays is also referred to as astraight angle A straight angle measures 180 degrees.
Angles – sides and vertexThere is another case where two rays can have a common endpoint.angleThis figure is called an .Some parts of angles have special names.SvertexThe common endpoint is called the ,and the two rays that make up the sides ofthe angle are called the sides of the angle.RvertexsideT
Naming AnglesThere are several ways to name this angle.S1) Use the vertex and a point from each side.SRTorTRSThe vertex letter is always in the middle.2) Use the vertex only.R1sideRvertexIf there is only one angle at a vertex, then theangle can be named with that vertex.3) Use a number.1T
AnglesAn angle is a figure formed by two noncollinear rays thathave a common endpoint.Symbols:DDefinitionof AngleDEFFEDE2EF2
Angles1) Name the angle in four ways.CABCACBA1B1B2) Identify the vertex and sides of this angle.vertex: Point Bsides:BAand BC
Angles1) Name all angles having W as their vertex.X12W12XWZY2) What are other names forXWYor1 ?YWX3) Is there an angle that can be namedNo!ZW?
Angle MeasureOnce the measure of an angle is known, the angle can be classifiedas one of three types of angles. These types are defined in relationto a right angle.Types of AnglesAobtuse angle90 mA 180AAright anglemA 90acute angle0 mA 90
Angle MeasureClassify each angle as acute, obtuse, or right.110 40 90 ObtuseRightAcute50 130 AcuteObtuse75 Acute
Adjacent AnglesWhen you “split” an angle, you create two angles.The two angles are calledadjacent anglesadjacent next to, joining.AB21 1 and 2 are examples of adjacent angles.They share a common ray.Name the ray that 1 and 2 have in common.CBD
Adjacent AnglesAdjacent angles are angles that:A) share a common sideB) have the same vertex, andC) have no interior points in commonDefinition ofAdjacentAnglesJR 1 and 2 are adjacentwith the same vertex R and21common side RMN
Adjacent AnglesDetermine whether 1 and 2 are adjacent angles.No. They have a common vertex B, butnocommon side21B1Yes. They have the same vertex G and acommon side with no interior points incommon.2GNLJ21No. They do not have a common vertex oracommon sideLNThe side of 1 isJNThe side of 2 is
Adjacent Angles and Linear Pairs of AnglesDetermine whether 1 and 2 are adjacent angles.No.12Yes.1X2DZIn this example, the noncommon sides of the adjacent angles form astraightline.linear pairThese angles are called a
Linear Pairs of AnglesTwo angles form a linear pair if and only if (iff):A) they are adjacent andB) their noncommon sides are opposite raysADefinition ofLinear PairsDB12 1 and 2 are a linear pair.BA and BD form AD 1 2 180
Linear Pairs of AnglesIn the figure, CM and CE are opposite rays.1) Name the angle that forms alinear pair with 1. ACEHTA21 ACE and 1 have a common side CAthe same vertex C, and opposite rays3 4CMCM and CE2) Do 3 and TCM form a linear pair? Justify your answer.No. Their noncommon sides are not opposite rays.E
Complementary and Supplementary AnglesTwo angles are complementary if and only if (iff)The sum of their degree measure is 90.EDADefinition ofComplementaryAnglesB30 60 FCm ABC m DEF 30 60 90
Complementary and Supplementary AnglesIf two angles are complementary, each angle is acomplement of the other. ABC is the complement of DEF and DEF is thecomplement of ABC.EABD30 C60 FComplementary angles DO NOT need to have a common sideor even the same vertex.
Complementary and Supplementary AnglesSome examples of complementary angles are shown below.75 15 HPm H m I 90Q40 m PHQ m QHS 9050 HSUTI60 Vm TZU m VZW 9030 ZW
Complementary and Supplementary AnglesIf the sum of the measure of two angles is 180, they form aspecial pair of angles called supplementary angles.Two angles are supplementary if and only if (iff) thesum of their degree measure is 180.DCDefinition ofSupplementaryAngles50 A130 BEm ABC m DEF 50 130 180F
Complementary and Supplementary AnglesSome examples of supplementary angles are shown below.H75 105 Im H m I 180Q130 50 HPSUV60 120 60 ZTm PHQ m QHS 180m TZU m UZV 180andWm TZU m VZW 180
Congruent AnglesmeasureRecall that congruent segments have the same .Congruentanglesalso have the same measure.
Congruent AnglesTwo angles are congruent iff, they have the samedegree measure.Definition ofCongruentAngles B V iff50 50 BVm B m V
Congruent AnglesarcsTo show that 1 is congruent to 2, we use .12To show that there is a second set of congruent angles, X and Z,we use double arcs.This “arc” notation states that: X ZXm X m ZZ
Vertical AnglesWhen two lines intersect, fourangles are formed.There are two pair of nonadjacent angles.vertical anglesThese pairs are called .4132
Vertical AnglesTwo angles are vertical iff they are twononadjacent angles formed by a pair ofintersecting lines.Vertical angles:Definition ofVerticalAngles413 1 and 32 2 and 4
Vertical AnglesVertical angles are congruent.Theorem 3-1VerticalAngleTheoremn2m 1 3314 2 4
Vertical AnglesFind the value of x in the figure:130 x The angles are vertical angles.So, the value of x is 130 .
Vertical AnglesFind the value of x in the figure:(x – 10) 125 The angles are vertical angles.(x – 10) 125.x – 10 125.x 135.
Congruent AnglesSuppose A B and m A 52.Find the measure of an angle that is supplementary to B.AB52 B 1 180 1 180 – B 1 180 – 52 1 128 1
Congruent AnglesGD11) If m 1 2x 3 and them 3 3x 2, then find them 3x 17; 3 37 A432BCEH2) If m ABD 4x 5 and the m DBC 2x 1, then find the m EBCx 29; EBC 121 3) If m 1 4x - 13 and the m 3 2x 19, then find the m 4x 16; 4 39 4) If m EBG 7x 11 and the m EBH 2x 7, then find the m 1x 18; 1 43
Once the measure of an angle is known, the angle can be classified as one of three types of angles. These types are defined in relation to a right angle. Types of Angles A right angle m A 90 acute angle 0 m A 90 A obtuse angle 90 m A 180 A Angle Measure
Aug 18, 2015 · Measure and classify angles. Identify and use congruent angles and the bisector of an angle. Discover relationships between special pair of angles. Vocabulary Degree, ray, angle, sides, vertex, interior, exterior, right angle, acute angle, obtuse angle, angle bisector. Adjacent angles, linear pair angles, vertical ang
1.6 Angle Pair Relationships 45 Finding Angle Measures In the stair railing shown at the right, 6 has a measure of 130 . Find the measures of the other three angles. SOLUTION 6 and 7 are a linear pair. So, the sum of their measures is 180 . m 6 m 7 180 130 m 7 180 m 7 50 6 and 5 are also a linear pair .
Before measuring an angle, it is helpful to estimate the measure by using benchmarks, such as a right angle and a straight angle. For example, to estimate the measure of the blue angle below, compare it to a right angle and to a straight angle. 90 angle 180 angle A right angle has a measure of 90
An angle is said to be an obtuse angle if it is greater than 90 but is less than 120 . Straight Angle An angle whose measure is 180 is a straight angle. This angle got its name as it forms a straight line. AOB is a straight angle. Reflex Angle A reflex angle
2] a pair of supplementary angles 3] a pair of complementary angles 4] a pair of adjacent angles 5] a pair of vertical angles 6] two right angles Write each pair of angles that you named above into the proper column of the table below. Angle Relationships
Herringbone gear pair is composed of two matched herringbone gears. It can be classified into two types: external herringbone gear pair and internal herringbone gear pair. Involute herringbone gear pair of external gearing with parallel axes It consists of two matched involute herringbone gears of external gearing.
Vertical Angles: Two angles whose sides form two pairs of opposite rays. Acute Angle: An angle less than 90 degrees. Obtuse Angle: An angle greater than 90 degrees. Right Angle: An angle equal to 90 degrees. Straight Angle: An angle equal to 180 degrees. Midpoint: The point that divide
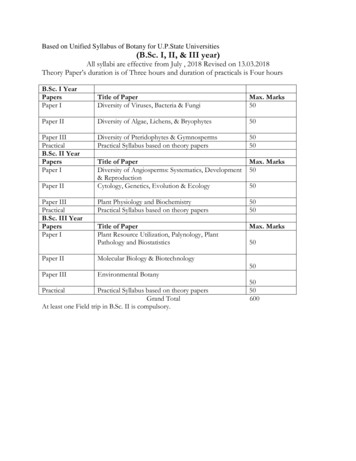
Diversity of Algae, Lichens, & Bryophytes 50 Paper III Diversity of Pteridophytes & Gymnosperms 50 Practical Practical Syllabus based on theory papers 50 B.Sc. II Year Papers Title of Paper Max. Marks Paper I Diversity of Angiosperms: Systematics, Development & Reproduction 50 Paper II Cytology, Genetics, Evolution & Ecology 50 Paper III Plant Physiology and Biochemistry 50 Practical Practical .