5.5 Parallel Lines And Transversals
EnglishSpanish5.5Parallel Lines and TransversalsHow can you useproperties of parallel lines to solve real-life problems?1ACTIVITY: A Property of Parallel LinesWork with a partner.Talk about what it means for two linesto be parallel. Decide on a strategy fordrawing two parallel lines.12cm111210345 967889Use your strategy to carefully drawtwo lines that are parallel.1011121361415516 7171841920321222322426parallellines2728in.2930Now, draw a third linethat intersects the twoparallel lines. This lineis called a transversal.25 121 2The two parallel lines andthe transversal form eightangles. Which of these angleshave equal measures?Explain your reasoning.4365transversalACTIVITY: Creating Parallel LinesWork with a partner.a. If you were buildingthe house in thephotograph, howcould you makesure that the studsare parallel to eachother?b. Identify sets ofparallel lines andtransversals in thephotograph.212Chapter 5Angles and Similarity78Studs
EnglishSpanish3ACTIVITY: Indirect MeasurementWork with a partner.Fa. Use the fact that two raysfrom the Sun are parallel toexplain why ABC and DEFare similar.b. Explain how to use similartriangles to find the heightof the flagpole.x ftSun’s rayCSun’s ray5 ftA3 ftBD36 ftE4. IN YOUR OWN WORDS How can you use properties of parallel linesto solve real-life problems? Describe some examples.5. INDIRECT MEASUREMENT PROJECT Work with a partner or in asmall group.a. Explain why the process in Activity 3 is called “indirect”measurement.b. Use indirect measurement to measure the height of somethingoutside your school (a tree, a building, a flagpole). Before goingoutside, decide what you need to take with you to do themeasurement.c. Draw a diagram of the indirect measurement process you used.In the diagram, label the lengths that you actually measuredand also the lengths that you calculated.Use what you learned about parallel lines and transversals tocomplete Exercises 3 – 6 on page 217.Section 5.5Parallel Lines and Transversals213
EnglishSpanishLesson5.5Lesson TutorialsLines in the same plane that do not intersect are called parallel lines.Lines that intersect at right angles are called perpendicular lines.Key Vocabularyperpendicular lines,p. 214transversal, p. 214interior angles,p. 215exterior angles,p. 215pIndicates linesand m areperpendicular.qIndicates lines pand q are parallel.mA line that intersects two or more lines is called a transversal. Whenparallel lines are cut by a transversal, several pairs of congruent anglesare formed.Corresponding AnglesStudy TiptWhen a transversal intersectsparallel lines, correspondingangles are congruent.Corresponding angleslie on the same sideof the transversal incorresponding positions.pqCorresponding anglesEXAMPLEa1Finding Angle MeasuresUse the figure to find the measures of (a) 1 and (b) 2.ba. 1 and the 110 angle are corresponding angles. They are congruent.So, the measure of 1 is 110 .110 2b. 1 and 2 are supplementary.1 1 2 180 t110 2 180 2 70 Definition of supplementary anglesSubstitute 110 for 1.Subtract 110 from each side.So, the measure of 2 is 70 .tExercises 7–9214Chapter 5Use the figure to find the measure ofthe angle. Explain your reasoning.1. 1Angles and Similarity2. 263 m12
EnglishSpanish2EXAMPLEaUsing Corresponding AnglesUse the figure to find the measures of the numbered angles.b 1: 1 and the 75 angle are vertical angles. They are congruent.2 75 1 35 64 7So, the measure of 1 is 75 .t 2 and 3: The 75 angle is supplementary to both 2 and 3.75 2 180 2 105 Definition of supplementary anglesSubtract 75 from each side.So, the measures of 2 and 3 are 105 . 4, 5, 6, and 7: Using corresponding angles, the measures of 4 and 6 are 75 , and the measures of 5 and 7 are 105 .m3. Use the figure to find the measuresof the numbered angles.Exercises 15–17When two parallel lines are cut by atransversal, four interior angles areformed on the inside of the parallel linesand four exterior angles are formed onthe outside of the parallel lines.t159 234576t13572p46q8 3, 4, 5, and 6 are interior angles. 1, 2, 7, and 8 are exterior angles.EXAMPLEClearance80 Sale3Standardized Test PracticeA store owner uses pieces of tape to paint a windowadvertisement. The letters are slanted at an 80 angle.What is the measure of 1?A80 B 100 C 110 D 120 1Because all of the letters are slanted at an 80 angle, the dashedlines are parallel. The piece of tape is the transversal.Using the corresponding angles, the 80 angle is congruent to the angle that issupplementary to 1, as shown.180 The measure of 1 is 180 80 100 . The correct answer is B .Section 5.5Parallel Lines and Transversals215
EnglishSpanish4. WHAT IF? In Example 3, the letters are slanted at a 65 angle.What is the measure of 1?Exercises 18 and 19Alternate Interior Angles and Alternate Exterior AnglesWhen a transversal intersects parallel lines, alternate interior anglesare congruent and alternate exterior angles are congruent.Study TiptAlternate interior anglesand alternate exteriorangles lie on oppositesides of the transversal.tppqqAlternate interior anglesEXAMPLE4Alternate exterior anglesIdentifying Alternate Interior and Alternate Exterior AnglesThe photo shows a portion of anairport. Describe the relationshipbetween each pair of angles.aba. 3 and 6 3 and 6 are alternateexterior angles.So, 3 is congruent to 6.12563478b. 2 and 7 2 and 7 are alternateinterior angles.So, 2 is congruent to 7.Exercises 20 and 21216Chapter 5In Example 4, the measure of 4 is 84 . Find the measure ofthe angle. Explain your reasoning.5. 3Angles and Similarity6. 57. 6
EnglishSpanishExercises5.5Help with Homework1. VOCABULARY Draw two parallel lines and a transversal. Label a pair ofcorresponding angles.2. WHICH ONE DOES NOT BELONG? Which statement does not belong with theother three? Explain your reasoning. Refer to the figure for Exercises 3 – 6.The measure of 2The measure of 5The measure of 6The measure of 86) 39 (- 3) 3 (- 9) 4 (- 1)9 (-In Exercises 3 – 6, use the figure.1 24 35 68 73. Identify the parallel lines.4. Identify the transversal.5. How many angles are formed by the transversal?tnm6. Which of the angles are congruent?Use the figure to find the measures of the numbered angles.17.8.ta9.b1 2b49 95 107 456t310. ERROR ANALYSIS Describe and correct the errorin describing the relationship between the angles.1bta2a 5 is congruent to 6.5611. PARKING The painted linesthat separate parking spacesare parallel. The measure of 1 is 60 . What is the measureof 2? Explain.12. OPEN-ENDED Describe two real-life situationsthat use parallel lines.Section 5.5Parallel Lines and Transversals217
EnglishSpanish13. PROJECT Draw two horizontal lines and a transversal on a pieceof notebook paper. Label the angles as shown. Use a pair ofscissors to cut out the angles. Compare the angles to determinewhich angles are congruent.1 24 35 68 714. REASONING Refer to the figure for Exercise 13. What is the leastnumber of angle measures you need to know in order to find themeasure of every angle? Explain your reasoning.Use the figure to find the measures of the numbered angles. Explain your reasoning.2 15.16.t1b24361 3a757 617.a46599 12ttb13 2a4 57 6bComplete the statement. Explain your reasoning.3 18. If the measure of 1 124 , then the measure of 4 19. If the measure of 2 48 , then the measure of 3 .4 20. If the measure of 4 55 , then the measure of 2 .a721. If the measure of 6 120 , then the measure of 8 .22. If the measure of 7 50.5 , then the measure of 6 .23. If the measure of 3 118.7 , then the measure of 2 b.32 68 41 5.24. RAINBOW A rainbow is formed when sunlight reflects off raindrops atdifferent angles. For blue light, the measure of 2 is 40 . What is themeasure of 1?2125. REASONING If a transversal is perpendicular to two parallel lines,what can you conclude about the angles formed? Explain.126. WRITING Describe two ways you can show that 1is congruent to 7.357218Chapter 5Angles and Similarityc
EnglishSpanishCRITICAL THINKING Find the value of x.27.28.cdcadbb50íxí115íxía29. OPTICAL ILLUSION Refer to the figure.a. Do the horizontal lines appear tobe parallel? Explain.b. Draw your own optical illusionusing parallel lines.mímí30.GoalGoal64í58íEvaluate the expression.31. 4 3a. Find the value of x.b. Can you still get the red puck in thegoal if x is increased by a little?by a lot? Explain.xí2The figure shows the anglesused to make a double bank shot in an airhockey game.SKILLS REVIEW HANDBOOK32. 5(2)2 633. 11 ( 7)2 934. 8 22 135. MULTIPLE CHOICE The volume of the cylinder is 20π cubic inches.What is the radius of the base? SKILLS REVIEW HANDBOOKA 1 inch B 2 inches C 3 inches D 4 inches 5 in.Section 5.5Parallel Lines and Transversals219
Section 5.5 Parallel Lines and Transversals 215 EXAMPLE 2 Using Corresponding Angles Use the fi gure to fi nd the measures of the numbered angles. 1: 1 and the 75 angle are vertical angles. They are congruent. So, the measure of 1 is 75 . 2 and 3: The 75 angle is supplementary to both 2 and 3. 7
1. Lines that do not intersect are parallel lines. 2. Skew lines are coplanar. 3. Transversal is a line that intersects two or more lines. 4. Perpendicular lines are intersecting lines. 5. If two lines are parallel to a third line, then the two lines are parallel. You have just tried describing parallel and perpendicular lines. In
3 Parallel and Perpendicular Lines Mathematical Thinking: Mathematically proficient students can apply the mathematics they know to solve problems arising in everyday life, society, and the workplace. 3.1 Pairs of Lines and Angles 3.2 Parallel Lines and Transversals 3.3 Proofs with Parallel Lines 3.4 Proofs with Perpendicular Lines 3.5 Slopes of Lines 3.6 Equations of Parallel and .
3 Parallel and Perpendicular Lines 3.1 Pairs of Lines and Angles 3.2 Parallel Lines and Transversals 3.3 Proofs with Parallel Lines 3.4 Proofs with Perpendicular Lines 3.5 Equations of Parallel and Perpendicular Lines Tree House (p. 130) Kiteboarding (p. 143) Crosswalk (p. 154) Bike Path (p. 161) Gymnastics (p. 130) Bi
126 Chapter 3 Parallel and Perpendicular Lines 3.1 Lesson WWhat You Will Learnhat You Will Learn Identify lines and planes. Identify parallel and perpendicular lines. Identify pairs of angles formed by transversals. Identifying Lines and Planes parallel lines, p. 126 skew lines, p. 126 parallel
3-7 Slopes of Parallel and Perpendicular Lines Parallel lines are lines in the same plane that never intersect. All vertical lines are parallel. Non-vertical lines that are parallel are precisely those that have the same slope and different y-intercepts. The graphs below are for the linear equations y 2x 5 and y 2x – 3.
perpendicular lines. . .And Why To write an equation that models part of a leaded glass window, as in Example 6 3-7 11 Slope and Parallel Lines Key Concepts Summary Slopes of Parallel Lines If two nonvertical lines are parallel, their slopes are equal. If the slopes of two distinct nonvertical lines are equal, the lines are parallel.
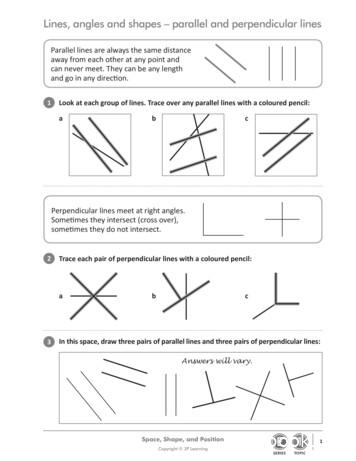
Look at each group of lines. Trace over any parallel lines with a coloured pencil: Lines, angles and shapes – parallel and perpendicular lines 1 2 3 Parallel lines are always the same distance away from each other at any point and can never meet. They can be any length and go in any direc on. ab c ab c Perpendicular lines meet at right angles.
Skew Lines Skew lines are lines that are and do not . In this diagram, planes R and W are parallel. DEand FGare lines. Perpendicular lines are not skew lines, because they're in the same . Parallel lines are skew lines,