An Introduction To Riemannian Geometry Matematikcentrum-PDF Free Download
Riemannian Geometry Dr Emma Carberry Semester 2, 2015 Lecture 15 [Riemannian Geometry - Lecture 15]Riemannian Geometry - Lecture 15 Riemann Curvature Tensor Dr. Emma Carberry September 14, 2015 Sectional curvature Recall that as a (1;3)-tensor, the Riemann curvature endomorphism of the Levi-Civita connection ris R(X;Y)Z r X(r YZ) r Y(r XZ .
inition of the Riemannian metric tensor which is of central importance to any analysis involving Riemannian geometry. Definition 1. (Riemannian metric) (do Carmo,1992) Given a smooth manifold M, a Riemannian metric on M assigns on each point p2Man inner product (i.e. a sym-metric, pos
MTH931 Riemannian Geometry II Thomas Walpuski Contents 1 Riemannian metrics4 2 The Riemannian distance4 3 The Riemanian volume form5 4 The Levi-Civita connection6 5 The Riemann curvature tensor7 6 Model spaces8 7 Geodesics10 8 The exponential map10 9 The energy functional12 10 The second variation formula13 11 Jacobi elds14 12 Ricci curvature16
complete riemannian manifolds of the same constant sectional curva-ture. In Chapter VIII one will find theorems of Myers and Synge, the Gauss-Bonnet formula and a result on complete riemannian manifolds of non-positive curvature. Then come the theorem of L.W. Green, which asserts that, on the two-dimensional real-projective space, a riemannian
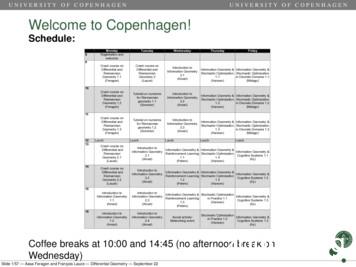
Differential and Riemannian Geometry 1.1 (Feragen) Crash course on Differential and Riemannian Geometry 3 (Lauze) Introduction to Information Geometry 3.1 (Amari) Information Geometry & Stochastic Optimization 1.1 (Hansen) Information Geometry & Stochastic Optimization in Discrete Domains 1.1 (M lago) 10 Cra
3.1.1 Riemannian Manifolds For most applications of di erential geometry, we are interested in manifolds in which all diagonal entries of the metric are positive. A manifold equipped with such a metric is called a Riemannian manifold. The simplest example is Euclidean space Rn which, in Cartesian coordinates, is equipped with the metric
1. Geometry, Riemannian. 2. Riemannian manifolds. I. Ebin, D. G. II. American Mathe-matical Society. III. Title. QA649.C47 2008 516.373—dc22 2007052113 Copying and reprinting. Individual readers of this publication, and nonprofit libraries acting for them, are permitted to make fair use of the material, such as to copy a chapter for use
Overdetermined PDE's in Riemannian Geometry Part III : general manifolds Alessandro Savo CUSO Mini-Course Workshop on Geometric Spectral Theory Neuchatel, June 19-20, 2017. Serrin problem on manifolds : existence We now consider compact domains in a general Riemannian manifold and study the Serrin problem: 8 :
Manifolds and Riemannian Geometry I M. do Carmo, Riemannian Geometry I J. M. Lee, manifold book series: I Introduction to Topological Manifolds I Introduction to Smooth Manifolds I Ri
Geometry in flat space: 1/17/17 “Do you have all these equations?” Before we begin with Riemannian manifolds, it’ll be useful to do a little geometry in flat space. Definition 1.1. Let V be a real vector space; then, an affine space over V is a set A with a simply transitive right V-action.
Riemannian Connection (do Carmo, 1992) and Riemannian Hessian (Absil et al., 2008) In an Euclidean space, A connection r amounts to the directional derivative of along at a point W, as r : lim t!0 W t t, where and are both vec-tor elds. This is the special case of a ne connec
Chapter 1: Introduction to differential and Riemannian geometry 3 1.2.1. Embedded Submanifolds Arguably the simplest example of a 2-dimensional manifold is the sphere S2. Relating to the previous example, when embedded in R3, we can view it as an idealized model for the surface of the earth
Riemannian Geometry with Applications to Mechanics and Relativity Leonor Godinho and Jos e Nat ario Lisbon, 2004. Contents Chapter 1. Differentiable Manifolds 3 1. Topological Manifolds 3 2. Differentiable Manifolds 9 3. Differentiable Maps 13 4. Tangent Space 15 5. Immersions and Embeddings 22File Size: 2MB
and Riemannian geometry (2003) , Providence (R. I.) : American mathematical society , cop. 2003 An introduction to differentiable manifolds and Riemannian geometry (2003) , William Munger Boothby, Amsterdam ; London ; New York [etc.] : Academic Press , cop. 2003 Osserman manifolds in semi-Rieman
notes: Eigenvalues in Riemannian geometry. By I. Chavel. Old and new aspects in Spectral Geometry. By M. Craiveanu, M. Puta and T. Ras-sias. The Laplacian on a Riemannian manifold. By S. Rosenberg. Local and global analysis o
Jan 10, 2006 · was Riemannian Geometry by Manfredo Perdigao do Carmo. Many other books are also mentioned in the notes. Since the professor handed out very good notes, I have made very few changes to these notes. 1. Chapter 1 January 10, 2006 Let Mn be a smooth manifold.
in these notes follow Do Carmo’s Riemannian Geometry. As per this text, the term di erentiable is taken to mean smooth. 2 Di erentiable Manifolds 2.1 Basic De nitions The rst step in our generalization of di erential geometry in Euclidean space is to take an arbitrary set that locally \lo
followed by a beginning course in Riemannian geometry (Chapters 5 – 8). This led to the idea of having a translation of the German . [14] M. do Carmo, “Riemannian Geometry”, Birkh auser, Boston-Basel-Berlin,1992. [15] J. A. S
attention, for the invitation t.o the conference Riemannian Geometry and Applica tions in Bra§ov, Romania, June 2007, and for many stimulating discussions. We also thank Jason Waller for the numerical confirmation of the conjecture. 1I
course. Instead, we will develop hyperbolic geometry in a way that emphasises the similar-ities and (more interestingly!) the many differences with Euclidean geometry (that is, the 'real-world' geometry that we are all familiar with). §1.2 Euclidean geometry Euclidean geometry is the study of geometry in the Euclidean plane R2, or more .
www.ck12.orgChapter 1. Basics of Geometry, Answer Key CHAPTER 1 Basics of Geometry, Answer Key Chapter Outline 1.1 GEOMETRY - SECOND EDITION, POINTS, LINES, AND PLANES, REVIEW AN- SWERS 1.2 GEOMETRY - SECOND EDITION, SEGMENTS AND DISTANCE, REVIEW ANSWERS 1.3 GEOMETRY - SECOND EDITION, ANGLES AND MEASUREMENT, REVIEW AN- SWERS 1.4 GEOMETRY - SECOND EDITION, MIDPOINTS AND BISECTORS, REVIEW AN-
Seminar on Riemannian Geometry Lukas Hahn July 9, 2015 1 Geodesics 1.1 Motivation The general idea behind the concept of geodesics is the generalisation of straight lines in Euclidian space to Riemannian manifolds. A geodesic will be a constantly paramatrized, smooth curve on the manifo
Corrections and additions for 2nd Edition of Riemannian Geometry I’d like to thank Victor Alvarez, Igor Belegradek, Gil Cavalcanti, Or Her-shkovits, Mayer Amitai Landau, Pablo Lessa, Ciprian Manolescu, Jiayin Pan, Jake Solo
In Lorenzi et al. (2015), the authors used Riemannian manifold techniques to estimate a model of normal brain ageing from MR images. The model was used to compute a time shift, called morphological age shift, which corresponds to the actual anatomical age of . Riemannian geometry, see Do Carmo
Assmc-r. This book provides an introduction to differential geometry, with prinicpal emphasis on Riemannian geometry . It covers the essentials, concluding with a chapter on the Yamaha problem, which shows what research in the Said looks like. It is a textb
Analytic Geometry Geometry is all about shapes and their properties. If you like playing with objects, or like drawing, then geometry is for you! Geometry can be divided into: Plane Geometry is about flat shapes like lines, circles and triangles . shapes that can be drawn on a piece of paper S
geometry is for its applications to the geometry of Euclidean space, and a ne geometry is the fundamental link between projective and Euclidean geometry. Furthermore, a discus-sion of a ne geometry allows us to introduce the methods of linear algebra into geometry before projective space is
Mandelbrot, Fractal Geometry of Nature, 1982). Typically, when we think of GEOMETRY, we think of straight lines and angles, this is what is known as EUCLIDEAN geometry, named after the ALEXANDRIAN Greek mathematician, EUCLID. This type of geometry is perfect for a world created by humans, but what about the geometry of the natural world?
Geometry IGeometry { geo means "earth", metron means "measurement" IGeometry is the study of shapes and measurement in a space. IRoughly a geometry consists of a speci cation of a set and and lines satisfying the Euclid's rst four postulates. IThe most common types of geometry are plane geometry, solid geometry, nite geometries, projective geometries etc.
P 6-8. Guide to Sacred Geometry - W ho Is the Course For? - The Program. P 9. Sacred Geometry: Eternal Essence - Quest For the Fundamental Dynamic P 10. W hat is Sacred Geometry? P 11. The PRINCIPLES of Sacred Geometry. P 13. Anu / Slip Knot & Sun's Heart (Graphic) P 14. History of Sacred Geometry. P 17. New Life Force Measure Sample Graphs .
rithms. Moreover, the new method inherits the detailed convergence analysis of the generic Riemannian trust-region method. Keywords: optimization on manifolds, trust-region methods, Newton’s method, symmetric generalized eigenvalue problem 1. Introduction Trust-region methods are wi
work/products (Beading, Candles, Carving, Food Products, Soap, Weaving, etc.) ⃝I understand that if my work contains Indigenous visual representation that it is a reflection of the Indigenous culture of my native region. ⃝To the best of my knowledge, my work/products fall within Craft Council standards and expectations with respect to
Riemannian geometry, complex (algebraic) geometry, PDE and analysis. IA paradigm is the case of complex dimension 1. A compact Riemann surface has an essentially unique metric of constant Gauss curvature. This is essentially the uniformisation theorem (for compact Riemann surfaces). IThe
DIFFERENTIAL GEOMETRY NOTES HAO (BILLY) LEE Abstract. These are notes I took in class, taught by Professor Andre Neves. I claim no credit to the originality of the contents of these notes. Nor do I claim that they are without errors, nor readable. Reference: Do Carmo Riemannian Geometry
Lecture 1 Notes on Geometry of Manifolds Lecture 1 Thu. 9/6/12 Today Bill Minicozzi (2-347) is filling in for Toby Colding. We will follow the textbook Riemannian Geometry by Do Carmo. You have to spend a lot of time on basics about manifolds, tensors, etc. and prerequisites like differential
2 Background on hyperbolic geometry Hyperbolic space is a non-Euclidean space with a negative constant sectional curvature and an underlying geometry that describes tree-like graphs with small distortions [46]. There exist four commonly-used models for hyperbolic spaces: Poincaré disk model, Lorentz model (hyperboloid
In this section, we recall some backgrounds on hyperbolic geometry, as well as some elementary operations of graph attention networks. Hyperbolic Geometry. A Riemannian manifold (M;g) of dimension nis a real and smooth manifold equipped with an inner product on tangent space g x: T xMT xM!R at each point x2M, where the tangent space T
These notes accompany my Michaelmas 2012 Cambridge Part III course on Dif-ferential geometry. The purpose of the course is to coverthe basics of differential manifolds and elementary Riemannian geometry, up to and including some easy comparison theorems. Time permitting, Penrose's incompleteness theorems of general relativity will also be .
4-DIMENSIONAL LOCALLY CAT(0)-MANIFOLDS WITH NO RIEMANNIAN SMOOTHINGS M. DAVIS, T. JANUSZKIEWICZ, and J.-F. LAFONT Abstract We construct examples of 4-dimensional manifolds M supporting a locally CAT(0)- metric, whose universal covers MQ satisfy Hruska’s isolated flats condition, and con- tain 2-dimensio
In summary, the main contributions of this work are: We propose an embedding method for Gaussians by mapping them diffeomorphically to Riemannian sym-metric spaces. We consider representing a 3D skeleton sequence by a set of SPD matrices that leads us to the study of statistics on Sym n. We