Transmission Lines And Power Flow Analysis
School of Engineering (SOE)University of St Thomas (UST)Transmission Lines andPower Flow AnalysisDr. Greg MowryAnnie SebastianMarian Mohamed1
School of EngineeringThe UST MissionInspired by Catholic intellectual tradition, theUniversity of St. Thomas educates studentsto be morally responsible leaders who thinkcritically, act wisely, and work skillfully toadvance the common good.http://www.stthomas.edu/mission/Since civilization depends on power, power is for the‘common good’!!2
School of EngineeringOutlineI. Background materialII. Transmission Lines (TLs)III. Power Flow Analysis (PFA)3
School of EngineeringI. Background Material4
School of EngineeringNetworks & Power Systems In a network (power system) there are 6 basicelectrical quantities of interest:1. Current𝑖 𝑡 𝑑𝑞/𝑑𝑡2. Voltage𝑣 𝑡 𝑑𝜑/𝑑𝑡3. Power𝑝 𝑡 𝑣 𝑡 𝑖 𝑡 𝑑𝑤𝑑𝑡(FL) 𝑑𝐸𝑑𝑡5
School of EngineeringNetworks & Power Systems 6 basic electrical quantities continued:4. Energy (work)w t 𝑡𝑝 5. Charge (q or Q)𝑞 𝑡 𝑡𝑖 𝜑 𝑡 𝑡𝑣 6. Flux𝜏 𝑑𝜏 𝑡𝑣 𝜏 𝑖 𝜏 𝑑𝜏𝜏 𝑑𝜏𝜏 𝑑𝜏6
School of EngineeringNetworks & Power Systems A few comments: Voltage (potential) has potential-energy characteristicsand therefore needs a reference to be meaningful; e.g.ground 0 volts I will assume that pretty much everything we discuss& analyze today is linear until we get to PFA7
School of EngineeringNetworks & Power Systems Linear Time Invariant (LTI) systems: Linearity:If𝑦1 𝑓 𝑥1&𝑦2 𝑓(𝑥2 )Then𝛼 𝑦1 𝛽 𝑦2 𝑓(𝛼 𝑥1 𝛽 𝑥2 )8
School of EngineeringNetworks & Power Systems Implications of LTI systems: Scalability:if 𝑦 𝑓(𝑥), then 𝛼𝑦 𝑓(𝛼𝑥) Superposition & scalability of inputs holds Frequency invariance:𝜔𝑖𝑛𝑝𝑢𝑡 𝜔𝑜𝑢𝑡𝑝𝑢𝑡9
School of EngineeringNetworks & Power Systems ACSS and LTI systems: Suppose: 𝑣𝑖𝑛 𝑡 𝑉𝑖𝑛 cos 𝜔𝑡 𝜃 Since input output, the only thing a linear system cando to an input is: Change the input amplitude: Vin VoutChange the input phase: in out10
School of EngineeringNetworks & Power Systems ACSS and LTI systems:These LTI characteristics along with Euler’s theorem𝑒 𝑗𝜔𝑡 cos 𝜔𝑡 𝑗 sin 𝜔𝑡 ,allow 𝑣𝑖𝑛 𝑡 𝑉𝑖 cos 𝜔𝑡 𝜃 to be represented as𝑣𝑖𝑛 𝑡 𝑉𝑖 cos 𝜔𝑡 𝜃 𝑉𝑖 𝑒 𝑗𝜃 𝑉𝑖 𝜃11
School of EngineeringNetworks & Power Systems In honor of Star Trek,𝑉𝑖 𝑒 𝑗𝜃 𝑉𝑖 𝜃is called a ‘Phasor’ 12
School of Engineering𝑉𝑖 𝑒 𝑗𝜃 𝑉𝑖 𝜃13
School of EngineeringNetworks & Power Systems ACSS and LTI systems: Since time does not explicitly appear in a phasor, anLTI system in ACSS can be treated like a DC systemon steroids with j ACSS also requires impedances; i.e. AC resistance14
School of EngineeringNetworks & Power Systems Comments: Little, if anything, in ACSS analysis is gained bythinking of j 1 (that is algebra talk) Rather, since Euler Theorem looks similar to a 2Dvector𝑒 𝑗𝜔𝑡 cos 𝜔𝑡 𝑗 sin 𝜔𝑡𝑢 𝑎𝑥 𝑏𝑦15
School of Engineering𝑒 𝑗90 cos 90 𝑗 sin 90 𝑗Hence in ACSS analysis,think of j as a 90 rotation16
School of EngineeringOperatorsv(t )V V dvdtj V vdtVj 17
School of EngineeringOhm’s Approximation (Law)Summary of voltage-current relationshipElementTime domainFrequency domainRv RiV RILdiv LdtV j LICdvi CdtV Ij C18
School of EngineeringImpedance & AdmittanceImpedances and admittances of passive elementsElementRImpedanceZ RLZ j LC1Z j CAdmittance1Y RY 1j LY j C18
School of EngineeringThe Impedance TriangleZ R jX18
School of EngineeringThe Power Triangle (ind. reactance)S P jQ18
School of EngineeringTriangles Comments: Power Triangle Impedance Triangle The cos( ) defines the Power Factor (pf)22
School of EngineeringReminder of Useful Network Theorems KVL – Energy conservation𝑣𝐿𝑜𝑜𝑝 0 KCL – Charge conservation𝑖𝑁𝑜𝑑𝑒 𝑜𝑟 𝑏𝑢𝑠 0 Ohm’s Approximation (Law) Passive sign Convention (PSC)23
School of EngineeringPassive Sign Convection – Power System24
School of EngineeringPSCSourceLoad or Circuit ElementPSource viPLoad viPSource PLoad25
School of EngineeringReminder of Useful Network Theorems Pointing Theorem𝑆 𝑉 𝐼 Thevenin Equivalent – Any linear circuit may berepresented by a voltage source and series Theveninimpedance Norton Equivalent – Any linear circuit my berepresented by a current source and a shunt Theveninimpedance26𝑉𝑇𝐻 𝐼𝑁 𝑍𝑇𝐻
School of Engineering (SOE)University of St Thomas (UST)Transmission Lines andPower Flow AnalysisDr. Greg MowryAnnie SebastianMarian Mohamed1
School of EngineeringII. Transmission Lines2
School of EngineeringOutline General Aspects of TLs TL Parameters: R, L, C, G 2-Port Analysis (a brief interlude) Maxwell’s Equations & the Telegraph equations Solutions to the TL wave equations3
School of Engineering4
School of Engineering5
School of EngineeringTransmission Lines (TLs) A TL is a major component of an electricalpower system. The function of a TL is to transport powerfrom sources to loads with minimal loss.6
School of EngineeringTransmission Lines In an AC power system, a TL will operateat some frequency f; e.g. 60 Hz. Consequently, 𝜆 𝑐/𝑓 The characteristics of a TL manifestthemselves when 𝝀 𝑳 of the system.7
School of EngineeringUSA example The USA is 2700 mi. wide & 1600 mi. fromnorth-to-south (4.35e6 x 2.57e6 m). For 60 Hz, 𝜆 3𝑒860 5𝑒6 𝑚 Thus 𝝀𝟔𝟎 𝑯𝒛 𝑳𝑼𝑺𝑨 and consequently thecontinental USA transmission system will exhibitTL characteristics8
School of EngineeringOverhead TL Components9
School of EngineeringOverhead ACSR TL Cables10
School of EngineeringSome Types of Overhead ACSR TL 038152156-801813127/7 Wires AAC Wasp Conductor Aluminium Electric Conductor.html
School of EngineeringTL Parameters12
School of EngineeringModel of an Infinitesimal TL Section13
School of EngineeringGeneral TL Parametersa. Series Resistance – accounts for Ohmic (I2R losses)b. Series Impedance – accounts for series voltage drops Resistive Inductive reactancec. Shunt Capacitance – accounts for Line-Charging Currentsd. Shunt Conductance – accounts for V2G losses due to leakagecurrents between conductors or between conductors and14ground.
School of EngineeringPower TL Parameters Series Resistance: related to the physical structure ofthe TL conductor over some temperature range. Series Inductance & Shunt Capacitance: producedby magnetic and electric fields around the conductorand affected by their geometrical arrangement. Shunt Conductance: typically very small soneglected.15
School of EngineeringTL Design ConsiderationsDesign ConsiderationsResponsibilitiesElectrical Factors1. Dictates the size, type and number of bundle conductors per phase.2. Responsible for number of insulator discs, vertical or v-shaped arrangement,phase to phase clearance and phase to tower clearance to be used3. Number, Type and location of shield wires to intercept lightning strokes.4. Conductor Spacing's, Types and sizesMechanical FactorsFocuses on Strength of the conductors, insulator strings and support structuresEnvironmental Factors Include Land usage, and visual impactEconomic FactorsTechnical Design criteria at lowest overall cost16
School of EngineeringTransmission Line ComponentsComponentsConductorsInsulatorsShield wiresSupport StructuresMade ofTypesACSR - Aluminum Conductor Steel ReinforcedAAC - All Aluminum ConductorAAAC - All Aluminum Alloy ConductorACAR - Aluminum Conductor Alloy ReinforcedAluminum replacedAlumoweld - Aluminum clad Steel ConductorcopperACSS - Aluminum Conductor Steel SupportedGTZTACSR - Gap-Type ZT Aluminum ConductorACFR - Aluminum Conductor Carbon ReinforcedACCR - Aluminum Conductor Composite ReinforcedPin Type InsulatorPorcelain, ToughenedSuspension Type Insulatorglass and polymerStrain Type InsulatorSteel, AlumoweldSmaller Cross section compared to 3 phase conductorsand ACSRWooden PolesLattice steel Tower, Reinforced Concrete PolesWood FrameSteel PolesLattice Steel towers.17
School of EngineeringACSR Conductor gWolfLynxPantherZebraDearMooseBersimisOverall Dia DC Resistance Current Capacity(mm)(ohms/km)(Amp) 75 .0559683635.040.0424299818
School of EngineeringTL Parameters - Resistance19
School of Engineering TL conductor resistance depends on factors suchas: Conductor geometry The frequency of the AC current Conductor proximity to other current-carrying conductors Temperature20
School of EngineeringResistanceThe DC resistance of a conductor at a temperature T is given by: 𝑹(𝑻) 𝝆𝑻𝒍𝑨where T conductor resistivity at temperature Tl the length of the conductorA the current-carry cross-sectional area of the conductorThe AC resistance of a conductor is given by: 𝑹𝒂𝒄 𝑷𝒍𝒐𝒔𝒔𝑰𝟐wherePloss – real power dissipated in the conductor in wattsI – rms conductor current21
School of EngineeringTL Conductor Resistance depends on: Spiraling:The purpose of introducing a steel core inside the strandedaluminum conductors is to obtain a high strength-to-weight ratio. Astranded conductor offers more flexibility and easier tomanufacture than a solid large conductor. However, the totalresistance is increased because the outside strands are larger thanthe inside strands on account of the spiraling.22
School of EngineeringThe layer resistance-per-length of each spirally woundconductor depends on its total length as follows:𝑹𝒄𝒐𝒏𝒅 𝝆𝑨𝟏 (𝝅𝟏)𝟐 Ω/m𝒑𝒄𝒐𝒏𝒅where𝑅𝑐𝑜𝑛𝑑 - resistance of the wound conductor (Ω)1 (𝜋p𝑐𝑜𝑛𝑑 1𝑃𝑐𝑜𝑛𝑑)2 - Length of the wound conductor (m)𝑙 𝑡𝑢𝑟𝑛2𝑟𝑙𝑎𝑦𝑒𝑟- relative pitch of the wound conductor (m)𝑙𝑡𝑢𝑟𝑛 - length of one turn of the spiral (m)2𝑟𝑙𝑎𝑦𝑒𝑟 - Diameter of the layer (m)23
School of Engineering Frequency: When voltages and currents change in time, current flow (i.e. currentdensity) is not uniform across the diameter of a conductor. Thus the ‘effective’ current-carrying cross-section of a conductor withAC is less than that for DC. This phenomenon is known as skin effect. As frequency increases, the current density decreases from that at thesurface of the conductor to that at the center of the conductor.24
School of Engineering Frequency cont. The spatial distribution of the current density in a particular conductor isadditionally altered (similar to the skin effect) due to currents in adjacentcurrent-carrying conductors. This is called the ‘Proximity Effect’ and is smaller than the skin-effect. Using the DC resistance as a starting point, the effect of AC on theoverall cable resistance may be accounted for by a correction factor k. k is determined by E&M analysis. For 60 Hz, k is estimated around 1.02𝑹𝑨𝑪 𝒌 𝑹𝑫𝑪25
School of EngineeringSkin Effect26
School of Engineering Temperature: The resistivity of conductors is a function of temperature. For common conductors (Al & Cu), the conductorresistance increases linearly with temperature𝜌 𝑇 𝜌0 1 𝛼 𝑇 𝑇𝑜 the temperature coefficient of resistivity27
School of EngineeringTL Parameters - Conductance28
School of EngineeringConductance Conductance is associated with power losses between theconductors or between the conductors and ground. Such power losses occur through leakage currents on insulatorsand via a corona Leakage currents are affected by: Contaminants such dirt and accumulated salt accumulated on insulators. Meteorological factors such as moisture29
School of EngineeringConductance Corona loss occurs when the electric field at the surface of aconductor causes the air to ionize and thereby conduct. Corona loss depends on: Conductor surface irregularities Meteorological conditions such as humidity, fog, and rain Losses due to leakage currents and corona loss are often smallcompared to direct I2R losses on TLs and are typically neglectedin power flow studies.30
School of EngineeringCorona loss31
School of EngineeringCorona loss32
School of EngineeringTL Parameters - Inductance33
School of EngineeringInductance is defined by the ratio of the total magnetic flux flowingthrough (passing through) an area divided by the current producingthat fluxΦ B d𝑠 𝐼 ,𝑂𝑏𝑠𝑒𝑟𝑣𝑎𝑡𝑖𝑜𝑛SΦL tance is solely dependent on the geometry of the arrangementand the magnetic properties (permeability ) of the medium.Also, B H where H is the mag field due to current flow only.34
School of EngineeringMagnetic Flux35
School of EngineeringInductance When a current flows through a conductor, a magnetic flux is setup which links the conductor. The current also establishes a magnetic field proportional to thecurrent in the wire. Due to the distributed nature of a TL we are interested in theinductance per unit length (H/m).36
School of EngineeringInductance - Examples37
School of EngineeringInductance of a Single WireThe inductance of a magnetic circuit that has a constant permeability (𝜇) can beobtained as follows:The total magnetic field Bx at x via Amperes law:𝜇𝑜 𝑥𝐵𝑥 𝐼 (𝑊𝑏/𝑚)22𝜋𝑟The flux d through slice dx is given by:𝑑Φ 𝐵𝑥 𝑑𝑎 𝐵𝑥 𝑙 𝑑𝑥 (𝑊𝑏)38
School of EngineeringThe fraction of the current I in the wire that links the area da dx l is:xNf r2The total flux through the wire is consequently given by:𝑟Φ 𝑟𝑑Φ 00𝑥𝑟2𝜇𝑜 𝑥 𝐼𝑙𝑑𝑥2𝜋𝑟 2Integrating with subsequent algebra to obtain inductance-per-m yields:𝐿𝑤𝑖𝑟𝑒 𝑠𝑒𝑙𝑓 0.5 10 7 (𝐻/𝑚)39
School of EngineeringInductance per unit length (H/m) for twoWires (Cables)To calculate the inductance per unit length (H/m) for two-parallelwires the flux through area S must be calculated.40
School of EngineeringThe inductance of a single phase two-wire line is given by:L 4 10 7lnDr′(H/m)where 𝑟 ′ 𝑟𝑥′ 𝑟𝑦′ 𝑒1 4𝑟𝑥41
School of EngineeringThe inductance per phase of a 3-phase 3 wire line with equal spacing is given by:La 2 10 7lnDr′(H/m) per phase42
School of EngineeringL of a Single Phase 2-conductor Line Comprisedof Composite Conductors43
School of Engineering𝐿𝑥 2 10 7𝐷𝑥𝑦ln𝐷𝑥𝑥where𝐷𝑥𝑦 𝑀𝑁𝑁𝑀𝐷𝑘𝑚𝑘 1𝑚 1′𝑎𝑛𝑑𝐷𝑥𝑥 𝑁2𝑁𝑀𝐷𝑘𝑚𝑘 1𝑚 1Dxy is referred to as GMD geometrical mean distance between conductors x & yDxx is referred to as GMR geometrical mean radius of conductor x44
School of EngineeringA similar Ly express exists for conductor y.LTotal Lx Ly(H/m)Great NEWs!!!!!Manufacturers often calculate these inductances for us forvarious cable arrangements 45
School of Engineering Long 3-phase TLs are sometimes transposed for positive sequence balancing. This is cleverly called ‘transposition’ The inductance (H/m) of a completely transposed three phase line may also becalculated46
School of Engineering𝐿𝑎 2 𝐷𝑒𝑞 10 73𝐷𝑒𝑞ln𝐷𝑠𝐷12 𝐷13 𝐷23La has units of (H/m)Ds is the conductor GMR for stranded conductors or r’ for solid conductors47
School of EngineeringIn HV TLs conductors are often arranged in one of thefollowing 3 standard configurations48
School of Engineering𝐿𝑎 2 10 7𝐷𝑒𝑞ln𝐷𝑆𝐿La again has units of (H/m)If bundle separation is large compared to the bundle size, then Deq the center-tocenter distance of the bundles2-conductor bundle: 𝐷𝑆𝐿 3-conductor bundle: 𝐷𝑆𝐿 4-conductor bundle: 𝐷𝑆𝐿 1694𝐷𝑆 𝑑2 𝐷𝑆 𝑑𝑑3 𝐷𝑆 𝑑𝑑𝑑 24𝐷𝑆 𝑑3𝐷𝑆 𝑑 24 1.091 𝐷𝑆 𝑑 349
School of EngineeringTL Parameters - Capacitance50
School of EngineeringQ CVC 𝑆 𝜀 𝐸 𝑑𝑠𝑙𝐸 𝑑𝑙𝜀RC 𝜎
School of EngineeringCapacitance A capacitor results when any two conductors are separated by an insulatingmedium. Conductors of an overhead transmission line are separated by air – which actsas an insulating medium – therefore they have capacitance. Due to the distributed nature of a TL we are interested in the capacitance perunit length (F/m). Capacitance is solely dependent on the geometry of the arrangement and theelectric properties (permittivity ) of the medium52
School of EngineeringCalculating Capacitance To calculate the capacitance between conductors wemust calculate two things: The flux of the electric field E between the two conductors The voltage V (electric potential) between the conductors Then we take the ratio of these two quantities53
School of Engineering Since conductors in a power system are typically cylindrical, westart with this geometry for determining the flux of the electricfield and potential (reference to infinity)54
School of EngineeringGauss’s Law may be used to calculate the electric field E and thepotential V for this geometry:𝑞𝐸𝑥 (𝑉/𝑚)2𝜋𝜀𝑥The potential (voltage) of the wire is found using this electric field:𝑉12𝑞 2𝜋𝜀𝐷2𝐷1𝑑𝑥𝑞𝐷2 𝑙𝑛𝑥2𝜋𝜀𝐷1𝑉𝑜𝑙𝑡𝑠The capacitance of various line configurations may be found using55these two equations
School of EngineeringCapacitance - Examples56
School of EngineeringCapacitance of a single-phase 2-Wire LineThe line-to-neutral capacitance is found to be:𝐶𝑛 2𝜋𝜀𝑙𝑛𝐷𝑟(𝐹/𝑚)The same Cn is also found for a 3-phase line-to-neutral arrangement
School of EngineeringThe Capacitance of Stranded Conductorse.g. a 3-phase line with 2 conductors per phase58
School of EngineeringDirect analysis yields𝐶𝑎𝑛2𝜋𝜀 �� 3𝐷𝑎𝑏 𝐷𝑎𝑐 𝐷𝑏𝑐59
School of EngineeringThe Capacitance of a Transposed Line60
School of EngineeringThe Capacitance of a Transposed Line𝐶𝑎𝑛 2𝜋𝜀𝐷𝑒𝑞𝑙𝑛 𝐷𝑆𝐶(𝐹/𝑚)where𝐷𝑒𝑞 3𝐷𝑎𝑏 𝐷𝑎𝑐 𝐷𝑏𝑐2-conductor bundle: 𝐷𝑆𝐶 3-conductor bundle: 𝐷𝑆𝐶 𝑟𝑑3𝑟 𝑑244-conductor bundle: 𝐷𝑆𝐶 1.091 𝑟 𝑑 361
School of EngineeringTLs ContinuedDr. Greg MowryAnnie SebastianMarian Mohamed1
School of EngineeringMaxwell’s Eqns. & theTelegraph Eqns.2
School of Engineering3
School of EngineeringMaxwell’s Equations & Telegraph Equations The ‘Telegrapher's Equations’ (or) ‘Telegraph Equations’ are apair of coupled, linear differential equations that describe thevoltage and current on a TL as functions of distance and time. The original Telegraph Equations and modern TL model wasdeveloped by Oliver Heaviside in the 1880’s. The telegraph equations lead to the conclusion that voltages andcurrents propagate on TLs as waves.4
School of EngineeringMaxwell’s Equations & Telegraph Equations The Transmission Line equation can be derived using Maxwell’sEquation. Maxwell's Equations (MEs) are a set of 4 equations that describeall we know about electromagnetics. MEs describe how electric and magnetic fields propagate, interact,and how they are influenced by other objects.5
School of EngineeringTime for History James Clerk Maxwell [1831-1879] was an Einstein/Newtonlevel genius who took the set of known experimental laws(Faraday's Law, Ampere's Law) and unified them into asymmetric coherent set of Equations known as Maxwell'sEquations. Maxwell was one of the first to determine the speed ofpropagation of electromagnetic (EM) waves was the same as thespeed of light - and hence concluded that EM waves and visiblelight were really descriptions of the same phenomena.6
School of EngineeringMaxwell’s Equations – 4 LawsGauss’ Law: The total of the electric flux through a closed surfaceis equal to the charge enclosed divided by the permittivity. Theelectric flux through an area is defined as the electric fieldmultiplied by the area of the surface projected in a planeperpendicular to the field.𝝆 𝑫 𝝐The ‘no name’ ME: The net magnetic flux through any closedsurface is zero. 𝑩 𝟎7
School of EngineeringFaraday’s law: Faraday's ‘law of induction’ is a basic law ofelectromagnetism describing how a magnetic field interacts over aclosed path (often a circuit loop) to produce an electromotive force(EMF; i.e. a voltage) via electromagnetic induction. 𝑩 𝑿𝑬 𝒕Ampere’s law: describes how a magnetic field is related to twosources; (1) the current density J, and (2) the time-rate-of-change ofthe displacement vector D. 𝑫 𝑿𝑯 𝑱 𝒕8
School of EngineeringThe Constitutive Equations Maxwell’s 4 equations are further augmented by the‘constitutive equations’ that describe how materials interact withfields to relate (B & H) and (D & E). For simple linear, isotropic, and homogeneous materials theconstitutive equations are represented by:𝑩 𝝁𝑯𝝁 𝝁𝒓 𝝁𝒐𝑫 𝜺𝑬𝝐 𝜺𝒓 𝜺𝒐9
School of EngineeringThe Telegraph Eqns.10
School of EngineeringTL Equations TL equations can be derived by two methods: Maxwell’s equations applied to waveguides Infinitesimal analysis of a section of a TL operating in ACSSwith LCRG parameters (i.e. use phasors) The TL circuit model is an infinite sequence of 2-portcomponents; each being an infinitesimally shortsegment of the TL.11
School of EngineeringTL Equations The LCRG parameters of a TL are expressed as perunit-length quantities to account for the experimentallyobserved distributed characteristics of the TL R’ Conductor resistance per unit length, Ω/m L’ Conductor inductance per unit length, H/m C’ Capacitance per unit length between TL conductors, F/m G’ Conductance per unit length through the insulating medium betweenTL conductors, S/m12
School of EngineeringThe Infinitesimal TL Circuit13
School of EngineeringThe Infinitesimal TL Circuit Model14
School of EngineeringTransmission Lines EquationsThe following circuit techniques and laws are used toderive the lossless TL equationsKVL around the ‘abcd’ loopKCL at node bVL L i tOLL for an inductorIC C v tOLC for a capacitorSet 𝑅′ 𝐺 ′ 0 for the Lossless TL case15
School of Engineering Apply KVL around the “abcd” loop: 𝑉(z) V(z z) 𝑅′ z 𝐼(𝑧)′I(z) 𝐿 z 𝑡 Divide all the terms by z : 𝑉 𝑧 𝑧 𝑉 𝑧 𝑧 𝑧 𝑅′ 𝐼 𝑧 𝐿′ 𝐼(𝑧) 𝑡 As z 0 in the limit and R’ 0 (lossless case): 𝑉(𝑧) 𝑧′ – 𝑅𝐼 𝑧 𝑽(𝒛) 𝒛 ′ 𝐼(𝑧) 𝐿 𝑡′ 𝑰(𝒛)–𝑳 𝒕(Eq.1)
School of Engineering Apply KCL at node b: 𝐼 (z) I(z z) 𝐺 ′ z 𝑉(𝑧 𝑧)′V(z z) 𝐶 z 𝑡 Divide all the terms by z: 𝐼 𝑧 𝑧 𝐼 𝑧 𝑧 𝑧 𝐺 ′ 𝑉 𝑧 𝑧 𝐶 ′ 𝑉(𝑧 𝑧) 𝑡 As z 0 in the limit and G’ 0 (lossless case): 𝐼(𝑧) 𝑧 – 𝐺 ′𝑉 𝑧 𝐶′ 𝑰(𝒛) 𝒛 –′ 𝑽(𝒛)𝑪 𝒕 𝑉(𝑧) 𝑡(Eq.2)
School of EngineeringSummarizing(Eqn. 1) 𝑉 𝑧 ,𝑡 𝑧(Eqn. 2) 𝐼 𝑧 ,𝑡 𝑧 ′ 𝐼 𝑧 ,𝑡𝐿 𝑡′ 𝑉 𝑧 ,𝑡𝐶 𝑡Equations 1 & 2 are called the “Coupled First Order Telegraph Equations”18
School of Engineering Differentiating (Eq.1) wrt ‘z’ yields: 2 𝑉(𝑧) 2 𝑧 –2′ 𝐼(𝑧)𝐿 𝑧 𝑡(Eq.3) Differentiating (2) wrt ‘t’ yields: 2 𝐼(𝑧) 𝑧 𝑡 –2′ 𝑉(𝑧)𝐶 𝑡 2(Eq.4) Plugging equation 4 into 3: 𝟐 𝑽(𝒛) 𝒛𝟐 𝟐 𝑽(𝒛) 𝑳′ 𝑪′ 𝒕𝟐(Eq.5)19
School of EngineeringReversing the differentiation sequence Differentiating (Eq.1) wrt ‘t’ yields: 2 𝑉(𝑧) 𝑧 𝑡 – 2 𝐼(𝑧)′𝐿 𝑡 2(Eq.6) Differentiating (2) wrt ‘z’ yields: 2 𝐼(𝑧) 2 𝑧 –2′ 𝑉(𝑧)𝐶 𝑧 𝑡(Eq.7) Plugging equation 6 into 7: 𝟐 𝑰(𝒛) 𝒛𝟐 𝟐 𝑰(𝒛) 𝑳′ 𝑪′ 𝟐 𝒕(Eq.8)20
School of EngineeringSummarizing(Eqn. 5) 2 V(z) z2(Eqn. 8) 2 I(z) z2 2 V(z) L′ C′ t2 2 I(z) L′ C′ 2 tEquations 5 & 8 are the v(z,t) and i(z,t) TL wave equations21
School of Engineering What is really cool about Equations 5 & 8 is that the solution to awave equation was already known; hence solving these equationswas straight forward. The general solution of the wave equation has the following form:f z, t f(z ut)where the ‘wave’ propagates with Phase Velocity 𝑢u ωβ ωω L ′ C′ 1L ′ C′22
School of Engineering The solution of the general wave equation, f(z,t), describes theshape of the wave and has the following form:f z, t f(z ut)orf z, t f(z ut) The sign of 𝑢𝑡 determines the direction of wave propagation: 𝑓 𝑧 𝑢𝑡 Wave traveling in the z direction 𝑓 𝑧 𝑢𝑡 Wave traveling in the –z direction23
School of EngineeringThe ‘ z’ Traveling WE Solution 𝑓 𝑧, 𝑡 𝑓(𝑧 𝑢𝑡) 𝑓 𝑧 𝑢𝑡) 𝑓(0 𝑧 𝑢𝑡 0 𝑧 𝑢𝑡24
School of Engineering The argument of the wave equation solution is called thephase. Hence the culmination of all of this is that, “Wavespropagate by phase change” One other key point, the form of the voltage WE andcurrent WE are identical. Hence solutions to each mustbe of the same form and in phase.25
School of EngineeringWave Equation z increases as t increase for a constant phase26
School of EngineeringTL Solutions27
School of EngineeringTL Wave Equations - Recap(Eqn. 5) 2 V(z) z2(Eqn. 8) 2 I(z) z2 2 V(z) L′ C′ t2 2 I(z) L′ C′ 2 tEquations 5 & 8 are the v(z,t) and i(z,t) TL wave equations28
School of EngineeringACSS TL Analysis Recipe29
School of EngineeringInitial Steps Typically we will know or are given the source voltage. Forexample, it could be:𝒗𝒔𝒔 𝒕 𝟏𝟎 cos 107 𝑡 30 𝒗𝒐𝒍𝒕𝒔 From the time function we form the source phasor & know thefrequency that the TL operates at (in ACSS operation):𝛚 𝟏𝟎𝟕 𝒔 𝟏𝑽𝒔𝒔 𝟏𝟎 𝒋𝟑𝟎𝒆𝒗𝒐𝒍𝒕𝒔30
School of EngineeringACSS TL Analysis Under ACSS conditions the voltage and current solutions to the WEmay be expressed as: 𝑉 𝑧, 𝑡 𝑅𝑒 𝑉0 𝑒 𝑗(𝜔𝑡 γ𝑧) 𝑉0 𝑒 𝑗(𝜔𝑡 γ𝑧) 𝑉 𝑧 𝑉0 𝑒 𝑗γ𝑧 𝑉0 𝑒 𝑗γ𝑧Time Harmonic Phasor Form I 𝑧, 𝑡 𝑅𝑒 𝐼0 𝑒 𝑗(𝜔𝑡 γ𝑧) 𝐼0 𝑒 𝑗(𝜔𝑡 γ𝑧) I 𝑧 𝐼0 𝑒 𝑗γ𝑧 𝐼0 𝑒 𝑗γ𝑧 𝑉 𝑗γ𝑧𝑒𝑍0 𝑉 𝑗γ𝑧𝑒𝑍0Time Harmonic Phasor Form
School of EngineeringACSS TL Analysis Recipe Step 1: Assemble 𝑍0 , 𝑍𝐿 , 𝑙, 𝑎𝑛𝑑 γ Step 2: Determine Г𝐿 Step 3: Determine 𝑍𝑖𝑛 Step 4: Determine 𝑉𝑖𝑛 Step 5: Determine 𝑉0 Step 6: Determine 𝑉𝐿 Step 7: Calculate 𝐼𝐿 and 𝑃𝐿32
School of EngineeringStep 1: Assemble 𝒁𝟎 , 𝒁𝑳 , 𝒍, , and 𝜸 Characteristic Impedance:𝒁𝒐 𝑳′𝑽 𝑽𝒐𝒐 𝑪′𝑰𝒐 𝑰𝒐 Load Impedance: 𝑽𝑳𝑽 𝑽𝟎𝟎𝒁𝑳 𝒁𝟎 𝑰𝑳𝑽𝟎 𝑽𝟎 Complex Propagation Constant: ( 0 for lossless TL)𝜸 𝜶 𝒋𝜷 𝒋𝝎 𝑳′ 𝑪′33
School of EngineeringAdditional Initial Results Phase velocity uP: Identity:𝛚𝐮𝐏 𝛃𝟏𝐋′ 𝐂 ′𝐋′ 𝐂 ′ 𝛍 𝛆34
School of EngineeringStep 2: Determine Г𝑳 Reflection Coefficient: Г𝐿 𝑉0 𝑉0 Г𝐿 𝑍𝐿 𝑍0𝑍𝐿 𝑍0 𝑧𝐿 𝑍𝐿𝑍0 𝐼0 𝐼0𝒛𝑳 𝟏Г𝑳 𝒛𝑳 𝟏 Reflection Coefficient at distance z (from load to source -l):Г 𝒛 𝜸𝒛𝑽 𝒐 𝒆 𝜸𝒛𝑽 𝒐 𝒆 Г𝑳 𝒆𝒋𝟐𝜷𝒛35
School of EngineeringAdditional Step-2 Results VSWR:𝟏 𝚪𝑳𝐕𝐒𝐖𝐑 𝟏 𝚪𝑳 Range of the VSWR:𝟎 𝑽𝑺𝑾𝑹 36
School of EngineeringStep 3: Determine 𝒁𝒊𝒏 Input Impedance:𝒁𝒊𝒏𝑽𝒊𝒏 𝒛 𝒍𝒁𝑳 𝒁𝟎 𝐭𝐚𝐧𝐡(𝜸𝒍) 𝒁𝟎𝑰𝒊𝒏 𝒛 𝒍𝒁𝟎 𝒁𝑳 𝒕𝒂𝒏𝒉(𝜸𝒍) For a lossless line tanh γl j tan(βl):𝒁𝒊𝒏𝒁𝑳 𝒋𝒁𝟎 𝒕𝒂𝒏(𝜷𝒍) 𝒁𝟎𝒁𝟎 𝒋𝒁𝑳 𝒕𝒂𝒏(𝜷𝒍) At any distance 𝑧1 from the load:𝒁𝑳 𝒋𝒁𝟎 𝐭𝐚𝐧(𝜷𝒛𝟏 )𝒁(𝒛𝟏 ) 𝒁𝟎𝒁𝟎 𝒋𝒁𝑳 𝒕𝒂𝒏(𝜷𝒛𝟏 )37
School of EngineeringStep 4: Determine 𝑽𝒊𝒏𝑽𝒊𝒏 𝑽𝑺𝑺𝒁𝒊𝒏 𝑽𝒊𝒏 𝒛 𝒍𝒁𝒊𝒏 𝒁𝑺38
School of EngineeringStep 5: Determine 𝑽𝟎 𝑉 𝑧 𝑉0 𝑒 𝑗𝛽𝑧 𝑉0 𝑒 𝑗𝛽𝑧 𝑉0 (𝑒 𝑗𝛽𝑧 Г𝐿 𝑒 𝑗𝛽𝑧 ) 𝑉𝑖𝑛 𝑉𝑇𝐿 𝑧 𝑙 𝑉0 (𝑒 𝑗𝛽𝑙 Г𝐿 𝑒 𝑗𝛽𝑙 )𝑽𝟎 𝑽𝒊𝒏 𝒋𝜷𝒍(𝒆 Г𝑳 𝒆 𝒋𝜷𝒍 )39
School of EngineeringStep 6: Determine 𝑽𝑳 Voltage at the load, VL:𝑽𝑳 𝑽𝑻𝑳 𝒛 𝟎 𝑽𝒐 𝟏 𝚪𝑳where:𝑽 𝟎𝑽𝒊𝒏 𝒋𝜷𝒍(𝒆 Г𝑳 𝒆 𝒋𝜷𝒍 )40
School of EngineeringStep 7: Calculate 𝑰𝑳 𝐚𝐧𝐝 𝑷𝑳𝑽𝑳𝑰𝑳 𝒁𝑳𝑷𝒂𝒗𝒈 𝑷𝑳 𝑰𝒏 𝒈𝒆𝒏𝒆𝒓𝒂𝒍,𝟏 𝟐𝑰𝑳 𝑹𝒆(𝒁𝑳 )𝟐𝑺𝑳 𝑽𝑳 𝑰 𝑳 𝑷𝑳 𝒋 𝑸𝑳41
School of EngineeringFinal Steps After we have determined VL in phasor form, we might beinterested in the actual time function associated with VL; i.e.vL(t). It is straight forward to convert the VL phasor back to thevL(t) time-domain form; e.g. suppose:𝑽𝑳 𝟓 𝒆 Then with 𝒋𝟔𝟎 𝒗𝒐𝒍𝒕𝒔𝛚 𝟏𝟎𝟕 𝒔 𝟏𝒗𝑳 𝒕 𝟓 cos 107 𝑡 60 𝒗𝒐𝒍𝒕𝒔42
School of EngineeringSurge Impedance Loading (SIL)Lossless TL with VS fixed for line-lengths /443
School of Engineering2-Port Analysis44
School of EngineeringTwo Port Network45
School of Engineering A two-port network is an electrical ‘black box’with two sets of terminals. The 2-port is sometimes referred to as a fourterminal network or quadrupole network. In a 2-port network, port 1 is often considered asan input port while port 2 is considered to be anoutput port.46
School of Engineering A 2-port network model is used tomathematically analyze electrical circuits byisolating the larger circuits into smaller portions. The 2-port works as “Black Box” with itsproperties specified by a input/output matrix. Examples: Filters, Transmission Lines,Transformers, Matching Networks, Small Signal47Models
School of EngineeringCharacterization of 2-Port Network 2-port networks are considered to be linear circuitshence the principle of superposition applies. The internal circuits connecting the 2-ports are assumedto be in their zero state and free of independent sources. 2-Port Network consist of two sets of input & outputvariables selected from the overall V1, V2, I1, I2 set: 2 Independent (or) Excitation Variables 2 Dependent (or) Response Variables48
School of EngineeringCharacterization of 2-Port NetworkUses of 2-Port Network: Analysis & Synthesis of Circuits and Networks. Used in the field of communications, control system, power systems, andelectronics for the analysis of cascaded networks. 2-ports also show up in geometrical optics, wave-guides, and lasers By knowing the matric parameters describing the 2-port network, it can beconsidered as black-box when embedded within a larger network.49
School of Engineering2-Port Networks & Linearity Any linear system with 2 inputs and 2 outputs can always beexpressed as:𝑦1𝑎11 𝑎21𝑦2𝑎12𝑎22𝑥1𝑥250
2-Port 48308/chapter-10-two-port-networks51
School of EngineeringReview A careful review of the preceding TL analysis reveals that all wereally determined in ACSS operation was Vin & Iin and VL & IL. This reminds us of the exact input and output form of any linearsystem with 2 inputs and 2 outputs which can always beexpressed as:𝑦1𝑎11 𝑎21𝑦2𝑎12𝑎22𝑥1𝑥2 52
School of EngineeringTransmission Line as Two Port NetworkI1 IinZSI2 IL AVS -BV2 VLV1 VinCD-SourceZL-2-Port NetworkLoad
School of EngineeringRepresentation of a TL by a 2-port network Nomenclature Used: VS – Sending end voltage IS – Sending end current VR – Receiving end Voltage IR – Receiving end current54
School of EngineeringRepresentation of a TL by a 2-port network Relation between sending end and receiving end is given as:𝑉𝑆 𝐴 𝑉𝑅 𝐵 𝐼𝑅𝐼𝑆 𝐶 𝑉𝑅 𝐷 𝐼𝑅 In Matrix Form𝑉𝑆𝐴 𝐶𝐼𝑆𝐵𝐷𝑉𝑅𝐼𝑅𝐴𝐷 𝐵𝐶 155
School of Engineering4 Cases of Interest Case 1: Short TL Case 2: Mediu
Transmission Line Components School of Engineering Components Made of Types Conductors Aluminum replaced copper ACSR - Aluminum Conductor Steel Reinforced AAC - All Aluminum Conductor AAAC - All Aluminum Alloy Conductor ACAR - Aluminum Conductor Alloy Reinforced Alumoweld - Aluminum clad
HVAC transmission lines such as greater power carrying capacity, the ability to connect asynchronous AC electric systems, precise control of electric power flow, and longer underground and undersea transmission lines. Typically, overall losses in HVDC transmission are 30-50% less than HVAC transmission [11]. Although HVDC incurs power losses
Waves on Transmission Lines 4 Waves on Transmission Lines After completing this section, students should be able to do the following. Name several transmission lines. Explain the di erence between phase shift and time delay of a sinusoidal signal. Evaluate whether the transmission line theory or circuit theory has to be
Vice President and Head of Sales, Siemens Rajesh Narayan General Manager & Head Transmission Line BU, Power Transmission & Distribution, L&T Rajesh Narayan Head, Transmission Lines, Power Transmission & Distribution, L&T Construction Giridharan P Application Manager, Grid and Power Quality System
Skew Lines Skew lines are lines that are and do not . In this diagram, planes R and W are parallel. DEand FGare lines. Perpendicular lines are not skew lines, because they're in the same . Parallel lines are skew lines,
1. Lines that do not intersect are parallel lines. 2. Skew lines are coplanar. 3. Transversal is a line that intersects two or more lines. 4. Perpendicular lines are intersecting lines. 5. If two lines are parallel to a third line, then the two lines are parallel. You have just tried describing parallel and perpendicular lines. In
This product provides a local flow indication and automatically signals the operator or PLC if flow is too high or too low. Uses of the Flow-Alert flow meter include: bearing lubrication, case drain verification, gun drill cooling, pump flow confirmation, etc. FLOW-ALERT FLOW SWITCH 6000 PSI / 414 BARS MAX GPM LPM OIL FIGURE 1- Flow-AlERt Flow .
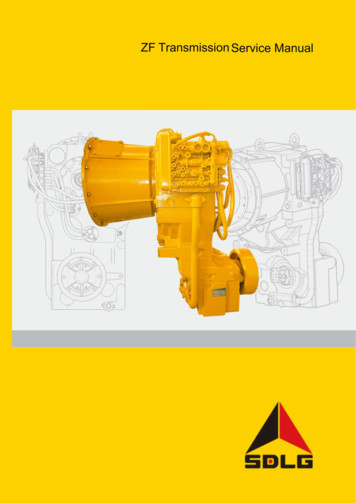
ZF Transmission Service Manual 5 2 Transmission System 2.1 Transmission Introduction 2.1.1 General Overview of the Transmission The ZF power gearshift transmission is composed of the hydraulic torque converter and rear-mounted countershaft transmission with multi-sheet friction clutch. The SDLG 938L、
IEC 61000-4-4 Electrical Fast Transients / Burst 2 kV Power Lines, 1 kV Interconnect Lines at 5kHz rate 2 kV Power Lines, 1 kV Interconnect Lines at 100kHz rate IEC 61000-4-5 Power Surge 2kV Power Lines 2kV Power Lines IEC 61000-4-6 Conducted Radio Frequency Immunity 3V at 0.15 – 80M